Rory
Closed Account
Here is a lovely shot of mountains in Oregon and Washington taken from the summit of South Sister in January 2007:

Source: https://www.flickr.com/photos/rhane/372202821/in/photostream/
The summit of South Sister is recorded as being ~10,360 feet above sea level (plus or minus 3 feet, depending on the source), and the GPS position of the photo, as well as I'm able to tell, is at or very close to 44.103500, -121.769250.
Here is the above image contrast-enhanced, cropped to the most relevant section, and with the mountain peaks labelled:

Elevations are taken from NAVD88. Other sources may vary up to 20 feet or so. These variations, however, make almost no difference to the results of the calculations, and zero difference to the conclusion.
This photo demonstrates that the Earth cannot be flat in a number of ways:
1. Everything in it aligns perfectly with a globe of radius ~3959 miles. Comparisons with globe-derived tools such as peakfinder, peakvisor, and Google Earth show no discrepancies.
2. A simple rule of perspective states that all points above one's eye level remain above eye level. If the earth were flat, Rainier should be the highest mountain in this photograph, with the viewer looking up to it. North Sister, however, which is lower than the camera, appears higher in the photograph than Mount Rainier.
3. If the earth were flat, Mount Rainier would be towering above Olallie Butte, rather than appearing at almost exactly the same apparent height.
4. Using simple pythagorean calculations, we can work out the apparent height order the mountains should appear in the photo if the earth was flat. They are (with their equivalent viewing angles):
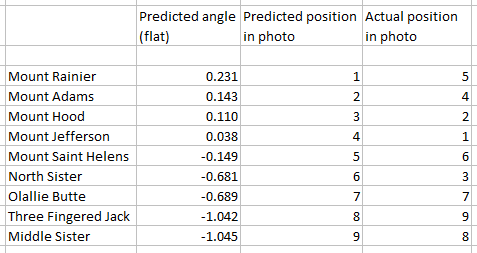
(A positive angle indicates above eye level - i.e., looking up - while a negative angle indicates looking down.)
5. It's also possible to use the predicted angles to demonstrate even further the flat earth model's incompatibility with this photograph. Not only are the positions inaccurate, but the intervals between the predicted angles are wildly divergent from what is seen. Rainier, Olallie, Saint Helens, and Middle Sister, for example, are all at about the same apparent height in the photograph, whereas the flat earth model predicts that they would be spread apart at a range of over 1.2 degrees.
6. By calculating the viewing angles to these mountains for a spherical earth model, we can see that they agree to a very high degree of accuracy:
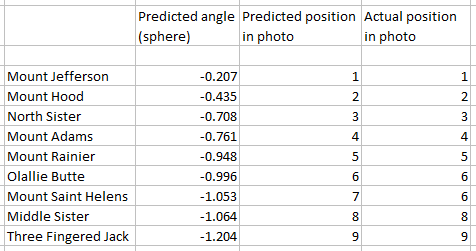
This time, the intervals between the angles agree with what is seen in the picture. The four mountains mentioned above - Rainier, Olallie, Saint Helens, and Middle Sister - are separated here by a difference of just 0.116 degrees, while the difference between Jefferson and Hood is about the same as the difference between Hood and North Sister.
The predicted order is also correct, apart from Olallie and Saint Helens, which are at exactly the same apparent height in the photo. This really is nitpicking, though: Olallie should probably appear only one or two pixels higher than Saint Helens, according to spherical calculations. A minute deviation of the camera from horizontal may explain this.
7. The angular size of the visible portions of the mountains are nothing like they should be on a flat earth, whereas they agree completely with a sphere earth.
8. Perhaps the most basic question for flat earth believers here is "where is eye level in this photo?" No matter where it is placed to align with two or more peaks, it will always be contradicted by others.
The rest of it is just gloss.
Source: https://www.flickr.com/photos/rhane/372202821/in/photostream/
The summit of South Sister is recorded as being ~10,360 feet above sea level (plus or minus 3 feet, depending on the source), and the GPS position of the photo, as well as I'm able to tell, is at or very close to 44.103500, -121.769250.
Here is the above image contrast-enhanced, cropped to the most relevant section, and with the mountain peaks labelled:
Elevations are taken from NAVD88. Other sources may vary up to 20 feet or so. These variations, however, make almost no difference to the results of the calculations, and zero difference to the conclusion.
This photo demonstrates that the Earth cannot be flat in a number of ways:
1. Everything in it aligns perfectly with a globe of radius ~3959 miles. Comparisons with globe-derived tools such as peakfinder, peakvisor, and Google Earth show no discrepancies.
2. A simple rule of perspective states that all points above one's eye level remain above eye level. If the earth were flat, Rainier should be the highest mountain in this photograph, with the viewer looking up to it. North Sister, however, which is lower than the camera, appears higher in the photograph than Mount Rainier.
3. If the earth were flat, Mount Rainier would be towering above Olallie Butte, rather than appearing at almost exactly the same apparent height.
4. Using simple pythagorean calculations, we can work out the apparent height order the mountains should appear in the photo if the earth was flat. They are (with their equivalent viewing angles):
(A positive angle indicates above eye level - i.e., looking up - while a negative angle indicates looking down.)
5. It's also possible to use the predicted angles to demonstrate even further the flat earth model's incompatibility with this photograph. Not only are the positions inaccurate, but the intervals between the predicted angles are wildly divergent from what is seen. Rainier, Olallie, Saint Helens, and Middle Sister, for example, are all at about the same apparent height in the photograph, whereas the flat earth model predicts that they would be spread apart at a range of over 1.2 degrees.
6. By calculating the viewing angles to these mountains for a spherical earth model, we can see that they agree to a very high degree of accuracy:
This time, the intervals between the angles agree with what is seen in the picture. The four mountains mentioned above - Rainier, Olallie, Saint Helens, and Middle Sister - are separated here by a difference of just 0.116 degrees, while the difference between Jefferson and Hood is about the same as the difference between Hood and North Sister.
The predicted order is also correct, apart from Olallie and Saint Helens, which are at exactly the same apparent height in the photo. This really is nitpicking, though: Olallie should probably appear only one or two pixels higher than Saint Helens, according to spherical calculations. A minute deviation of the camera from horizontal may explain this.
7. The angular size of the visible portions of the mountains are nothing like they should be on a flat earth, whereas they agree completely with a sphere earth.
8. Perhaps the most basic question for flat earth believers here is "where is eye level in this photo?" No matter where it is placed to align with two or more peaks, it will always be contradicted by others.
The rest of it is just gloss.
Last edited: