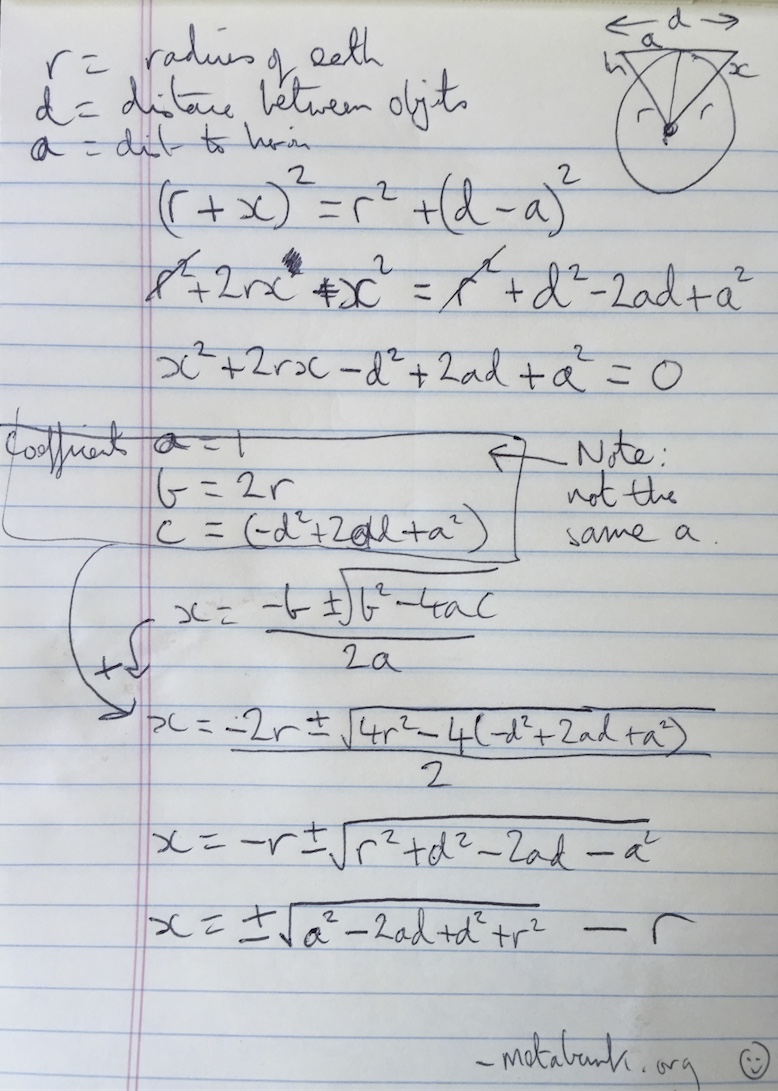Earth's Curve Calculator

[Mick West]

https://www.metabunk.org/curve/

To use it you just put in the distance to the object in miles and your height above the surface in feet.


The Metabunk curve calculator was written to provide a simple answer to the question: "how much of a distant object is hidden by the curve of the earth from a certain distance and view position". The following diagram is an exaggerated look at the numbers involved.

There's a version of this diagram on the calculator page that you can move around.

Notice there are two sets of results given, "Results ignoring refraction" and "With Standard Refraction".

The results ignoring refraction are the results if we ignore the effects of the air (the atmosphere) between the viewer (the eye position) and the target object.

When light passes though air that varies in density then it gets bent towards the higher density air. Normally air gets less dense as you go higher (think of thin air at the top of a mountain). When the light is bent down then it makes things look higher than they are. It can also bend the light around the curve of the earth.

This has the effect of (visually) flattening the Earth a bit, and we can use a hand rule of thumb (used by surveyors for 100+ years) of just doing the same calculation with a larger (7/6x) radius earth. That give you the second set of numbers.

In reality the "ignoring refraction" numbers are almost always going to be too small. The atmosphere always exists, and it's always refracting. On average it's going to give you the "standard refraction" values. However there's going to be considerable variations. "Standard" here refers to the international standard atmosphere which is simply an average state of the atmosphere used as a baseline. It's like there's a "standard" temperature of 66°F/19°C. Again that's an average, over the whole year. On some days it's only going to be 66°F for a few minutes, some days (and/or locations) it's never going to be that value.

So the "standard refraction" is just a typical value. Actual observations will vary.

Once you've got the results, you can save them by clicking on "Permalink

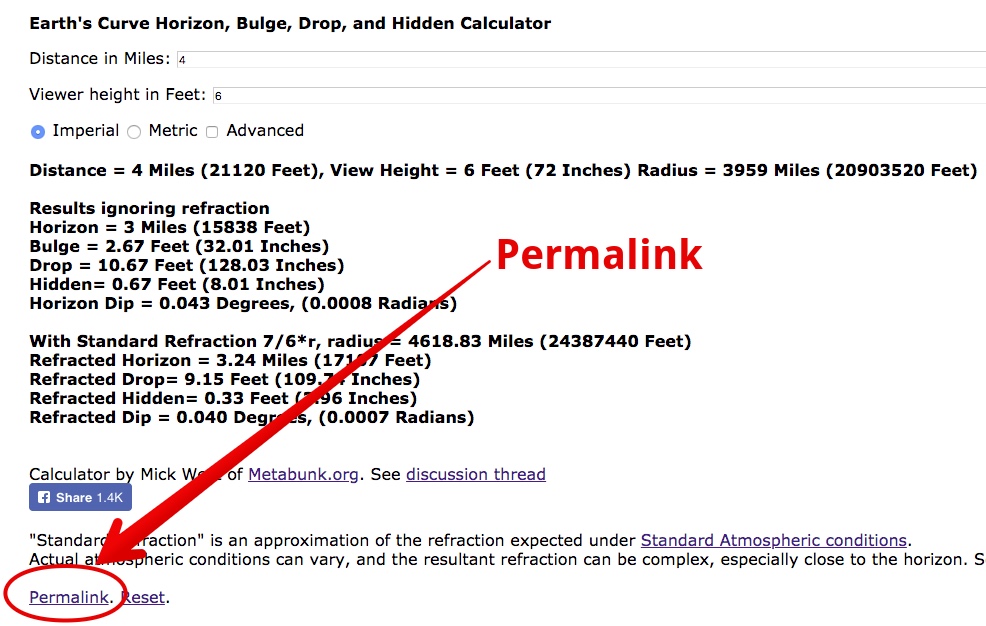
then copy the URL from the browser for sharing. It will look something like:
https://www.metabunk.org/curve/?d=4&h=6&r=3959&u=i&a=n&fd=60&fp=3264

You don't have to click on "permalink" again, the URL will update automatically.

There's also an "Advanced" version (click the "Advanced" checkbox just above the results). This gives you more options, and shows more results and the underlying math.



This calculator arose from discussion in this thread:
https://www.metabunk.org/earth-curv...bunking-flat-concave-earth.t6042/#post-149557

 

[Mick West]

It can be represented as a relatively short single equatioin,

amount obscured = r/cos( d/r - asin(sqrt(h*(2*r+h)) / (r+h) ) ) - r

Where h = height of the observer, and d = distance between points (as a great circle), and the trig functions use radians

I've updated the calculator.
https://www.metabunk.org/curve/

Today someone asked me about this formula, which I'd just extracted from here. I could not remember how I got it (four years ago), so I re-derived it from scratch. In doing this I realized it could be much simpler expressed (and explained) as

r/cos( d/r - acos(r / (r+h) ) ) - r

 

[Mick West]

Something that might come up regarding the calculator is if it makes a difference where on the Earth you are, due to the ellipsoidal shape of the Earth. A few years ago I investigated this, and found it did not make a significant difference. Details at:
https://www.metabunk.org/earth-curv...ipsoid-geoid-models-for-visibility-etc.t7954/


(just re-linking here, as this is the thread linked from the calculator)

 

[Mick West]

I also realized that in my original diagram:

The line labeled "Distance" is the chord distance, but the calculator uses the line-of-sight distance (from "Eye" to the intersection with the target).

I've updated the diagram to show the three ways the distance can be measured

Distance1 = Line of sight, from the eye/camera, grazing the horizon, to the target
Distance2 = Arc distance or surface distance. From water level beneath the eye to water level beneath the target
Distance3 = straight line between the Distance2 endpoints.

I've clarified the text under "advanced" and added a calculation based on Distance3:

Hidden3 = r/cos( 2*asin(d/(2*r)) - acos(r / (r+h) ) ) - r

since d2 = 2 * r * asin(d3/2r)

 

[Mick West]

For reference.

The second pages is a derivation of x directly from d3, which you can also get by substituting the previous equation of d2 in terms of d3.

And here's the original derivation of x from d1 (and h and r, of course).

I'd used the D1 method simply because I'm happier with pythagoras than with trig. But really r/cos( d/r - acos(r / (r+h) ) ) - r is such an elegant formula I wish I'd gone with that.

Bottom line though, there's no real difference up to 100 miles. And it's not like one method is more accurate than another, it depends on what you are measuring as d.

 

[Rory]

Ay, I played with the different ways of calculating the distance and the variations in the ellipsoid and found it's just a few feet here and there - usually around the height of a tree, even over a hundred miles or so.

I did add a step to calculate radius from latitude, which was quite nice. This is the equation for that for excel:

=SQRT(((3963.19059^2*COS(RADIANS(C5)))^2+(3949.90276^2*SIN(RADIANS(C5)))^2)/((3963.19059*COS(RADIANS(C5)))^2+(3949.90276*SIN(RADIANS(C5)))^2))

(C5=latitude)

Things I'd like to see on the calculator would be:

• The addition of an obstruction calculator (yes/no tick box for "is there an obstruction?")
• Predicted visible amount outputted, in addition to hidden
• Variable refraction option (say plus or minus a percentage from 'standard')
• The refracted results promoted above non-refracted (or non-refracted removed, or perhaps a simple check box for with/without refraction)
• Outputs for predicted hidden/visible amounts for the flat earth 'notion' (generally only required when there's an obstruction, but perhaps always good to have it there in black and white: e.g., 'Predicted hidden amount: ZERO')

 

[Bobby Shafto]

I also realized that in my original diagram:

[snip]

The line labeled "Distance" is the chord distance, but the calculator uses the line-of-sight distance (from "Eye" to the intersection with the target).

I just noticed this today. Was wondering if it'd been there all along and I had just not been observant.

On a tangential topic, can I ask what value "the bulge" is to anything?

I've been contemplating a presentation that deprecates the bulge (sagitta) in relation to calculations of earth curvature since I can't find any use for it. If anything, it fosters the notion that "the bulge" is what is obscuring, but that's only true when the horizon happens to coincide with it. Otherwise, I think it just contributes to the erroneous concept of the earth "rising up" like a wall or mound between observer and another point on the sphere.

I've been wanting to address this "amount of curvature" terminology that is so rampant among globe busting, and the incessant conflation by those who think "the drop" and amount hidden are the same thing and that "the bulge" is what contributes to amount hidden. "Drop" is useful, but "bulge?"

If I'm wrong, what am I missing?

 

[Mick West]

On a tangential topic, can I ask what value "the bulge" is to anything?
...
I've been wanting to address this "amount of curvature" terminology that is so rampant among globe busting, and the incessant conflation by those who think "the drop" and amount hidden are the same thing and that "the bulge" is what contributes to amount hidden. "Drop" is useful, but "bulge?"

I think it's perhaps useful to get a sense of scale. In the default, the "drop" is 10.67 feet, but the bulge is only 2.67 feet.

I've not really seen anyone confused by it though.

 

[Bobby Shafto]

I've repeatedly encountered globe "debunking" that uses height of the bulge as the obstruction and amount of "drop" what "should be hidden."

Drop amount, at least, is useful for calculating amount of declination something in the distance will show compared to across a plane.

Those with a propensity to misunderstand globe geometry misunderstand "the bulge" to be something that appears to rise before you, like a mound, hill or wall. Only if you were trying to sight along the chord would the bulge seem to rise, but who intuitively tries to use the chord as the sight line?

It's just a campaign I'm on to wean people away from "the bulge" since it doesn't contribute to the geometry of "what should be seen or not" at all. The horizon is the obstruction. Not the bulge. Unlike "drop," I can't find any usefulness for the "bulge" other than for flat earthers to misuse it.

Eventually, I'll publish my pitch (video) that we dispense with the "bulge." Just thought I'd voice it and see what anyone here thinks. Not a high priority thing, obviously. And yours and Walters calculators are so useful. I probably should pay you a subscription fee given the amount of times I rely on it.

 

[Bobby Shafto]

Yay. I see "bulge" has been replaced with "sagitta." May or may not be due to my input, but glad to see it nonetheless. Doubt it'll have any influence on the erroneous notion that the "bulge" (or sagitta) is responsible for obscuring line of sight, but it's a start.

 

[Mick West]

May or may not be due to my input

It was you. I was doing a general revamp, still a work in progress. I'm also going to add an angular size version

 

[deirdre]

It was you. I was doing a general revamp, still a work in progress. I'm also going to add an angular size version

hmm. noone knows what a sagitta is. i've never heard of that word in my life. you should at least put "bulge" in parenthesis next to it.

 

[Mick West]

hmm. noone knows what a sagitta is. i've never heard of that word in my life. you should at least put "bulge" in parenthesis next to it.

That's kind of the point though. The "bulge" sounds like it's an important number ("a 12-foot bulge of water") but it's not really relevant to any of the computations.

Still, there's a potential for confusion. Maybe a note.

 

[DavidB66]

If there is a general revamp of the calculator I suggest there should be a look at the 'horizon curve' feature. At present this uses a default field-of-view of over 90 degrees, which is so wide as to be irrelevant for most purposes. It may be based on the maximum field of view of the (single) human eye, which is said to be about 95 degrees, but much of that is in peripheral vision, which the observer is only vaguely aware of. If it is applied to a camera, a shot with such a wide fov would probably show major lens distortion. A default of, say, 70 degrees might be more useful.
The calculator also allows even wider fov's, up to 180 degrees and beyond. I notice that around 180 degrees the results seem to go mad. 'Horizon Curve Angle' (v1 or v2) suddenly jumps up to 90 degrees for an fov of 180, whereas Horizon Curve Fraction and Horizon Curve Pixels behave more sensibly. I don't even know what an fov of 180 degrees would mean for this purpose. Since the observer's viewpoint is always somewhere above the surface, the angle subtended at the viewpoint between two points on the horizon can never go up to 180 degrees. Unless there is a practical application for very high fov's, I suggest there might be a cutoff at, say, 100 degrees. I note that Walter Bislin's calculator/simulator seems to have a default fov of 65 degrees, and doesn't go beyond 92 degrees. (It is possible to enter higher values, but this doesn't seem to increase the visible curvature.)

 

[Mick West]

At present this uses a default field-of-view of over 90 degrees

Where are you getting that? The default FOV is 60:

 

[DavidB66]

Where are you getting that? The default FOV is 60:
View attachment 36689

That's odd. I have the calculator bookmarked here: https://www.metabunk.org/curve/?d=1&h=100000&r=3959&u=i&a=a&fd=94.4&fp=1920

which shows a default FoV of 94.4 degrees. But several of the other defaults are also different. Maybe I just bookmarked an obsolete version that is somehow still accessible? [Added: or the settings are just some that I once searched for and unintentionally got 'frozen into' the bookmarked version. But I can't remember or imagine using that particular set of settings. For example, to the best of my memory I have never even touched the 'image width' setting. ]

 

[Mick West]

That's odd. I have the calculator bookmarked here: https://www.metabunk.org/curve/?d=1&h=100000&r=3959&u=i&a=a&fd=94.4&fp=1920

which shows a default FoV of 94.4 degrees. But several of the other defaults are also different. Maybe I just bookmarked an obsolete version that is somehow still accessible? [Added: or the settings are just some that I once searched for and unintentionally got 'frozen into' the bookmarked version. But I can't remember or imagine using that particular set of settings. For example, to the best of my memory I have never even touched the 'image width' setting. ]

Yeah, you just want:
https://www.metabunk.org/curve/

Otherwise, it will read in the parameters from the URL. You get that URL when you click on "Permalink", you can reset them with "Reset"

 

[Rory]

That's kind of the point though. The "bulge" sounds like it's an important number ("a 12-foot bulge of water") but it's not really relevant to any of the computations.

Still, there's a potential for confusion. Maybe a note.

"Sagitta" is good. Like you say, "bulge" isn't really relevant, and makes people think there's a hill of water. If they can't understand the word "sagitta", but want to, they can always take five seconds to google it, and then they'll learn something, and maybe even go on from there to learn something else.

I like how the "standard refraction" comes first now, and is highlighted. And the "no refraction" has been termed "geometric".

 

[deirdre]

That's kind of the point though. The "bulge" sounds like it's an important number ("a 12-foot bulge of water") but it's not really relevant to any of the computations.

the curve of the ball vs a flat plane isnt relevant to computations?

 

[Mick West]

the curve of the ball vs a flat plane isnt relevant to computations?

The sagitta isn't used in the computations. It's a side effect.

The curve of the ball is defined by the radius. So we use the radius in the calculation.

The bulge height is the height in the middle, but this height is not the height of the horizon. It's not a height that obscures anything

See where the horizon is, above? The Sagitta is "related" in the sense that it's also calculated using the radius. But it's not really useful in calculating the hidden amount.

 

[deirdre]

The bulge height is the height in the middle, but this height is not the height of the horizon. It's not a height that obscures anything

i know because you have a big green X marked "horizon", which is quickly showing me the horizon is not the same as the bulge/sagitta. In your OP pic i see the horizon is past the bulge.

But if the sagitta is irrelevant to the calculations, then why even have that marked? (although unmarked, or marked sagitta, pretty sure all the non mathematicians of the world are still going to call it the bulge.. so if you guys like sagitta better that's cool)

 

[deirdre]

ps. the dots on all my browsers are red

 

[Rory]

Ditto. I'm guessing they used to be blue?

Did you notice the one that says "= Draggable" is also draggable. That's cool.

I've got a little j under my green line (line of sight to horizon). You?

 

[Mick West]

Ditto. I'm guessing they used to be blue?

Did you notice the one that says "= Draggable" is also draggable. That's cool.

I've got a little j under my green line (line of sight to horizon). You?

Fixed!

 

[Mick West]

Spurred on by @Rory's angular shenanigans, I've added a purely angular-size based calculation of the hidden amount.

This, of course, is in part to answer some critics of curve calculators in the FE community who claim the curve calculator ignores angular size. So I worked out equations that ignore things like calculating the drop or the horizon distance, and instead "simply" calculate the angular size of the portion of the target that is hidden.

It's somewhat pointless, as the end result is the same. It also might not be understandable if you didn't understand the old way. However, that's the math, and it can be verified.

Like before, there's three important numbers:

r = radius of the Earth
h = viewer height
d = distance to target

d here is Distance2 on the calculator diagram, i.e. the distance along the surface of the earth.

The dip to the horizon is just a very simple acos(r/(r+h))

The dip to the base of the target is more complicated, as we don't have a right angled triangle, so we have to use the Sine Rule and the Cosine Rule. Fun high school math
https://www.mathsisfun.com/algebra/trig-solving-triangles.html

1. The angles always add to 180°:
A + B + C = 180°

When you know two angles you can find the third.


2. Law of Sines (the Sine Rule):

When there is an angle opposite a side, this equation comes to the rescue.

Note: angle A is opposite side a, B is opposite b, and C is opposite c.



3. Law of Cosines (the Cosine Rule):

This is the hardest to use (and remember) but it is sometimes needed
to get you out of difficult situations.

It is an enhanced version of the Pythagoras Theorem that works
on any triangle.

So we just use the law of cosines to find the length of the line from the viewer to the base of the target

EyeToBase = sqrt(r^2 + (r+h)^2 - 2*(r+h)*r*Cos(d/r))

Then the law of sines to get the dip angle of the base

BaseDip = PI/2 - Asin(r*Sin(d/r)/EyeToBase)

In code

var r = 3959*5280; // radius of the earth in feet
var HorizonDip = Math.acos(r/(r+h))
var EyeToBase = Math.sqrt(r**2 + (r+h)**2 -2*r*(r+h)*Math.cos(d/r))
var K = Math.asin(r*Math.sin(d/r)/EyeToBase)
var BaseDip = Math.PI/2 - K;
var AngularHidden = BaseDip - HorizonDip

(Note: all angles are in radians. If degrees are used then d/r should be d/r*180/PI)

 

[rhodes0606]

Today someone asked me about this formula, which I'd just extracted from here. I could not remember how I got it (four years ago), so I re-derived it from scratch. In doing this I realized it could be much simpler expressed (and explained) as

r/cos( d/r - acos(r / (r+h) ) ) - r

Hi Mick, What would you say is the standard error for the calculation provided here? Since you were able to find the average for the atmospheric values, could we also determine the highest and lowest and get some resemblance of a standard deviation? I think that would be helpful to report. Just a thought. Thank you for doing all the hard work to provide such a great tool!

 

[Mathias]

Shouldn't "Geometric Horizon Dip" and "Angle between eye level and the horizon" be the same value? They are the same when using imperial but when using metric the calculator gives two different calues.

 

[Mick West]

Shouldn't "Geometric Horizon Dip" and "Angle between eye level and the horizon" be the same value? They are the same when using imperial but when using metric the calculator gives two different calues.

Thanks! I'd neglected to program the metric conversion for the new calculations, so it was using the Earth's radius in feet. Now fixed.

 

[Mick West]

Hi Mick, What would you say is the standard error for the calculation provided here? Since you were able to find the average for the atmospheric values, could we also determine the highest and lowest and get some resemblance of a standard deviation?

I didn't "find the average," I used a rule of thumb based on the standard atmosphere, which assumes a certain temperature and pressure at sea level, and then assumes a constant lapse rate (i.e the rate of decrease with height) for both temperature and pressure.

That's a reasonable approximation for many uses, especially peak-to-peak surveying. But in the real world the diurnal (day-night) heating and cooling of the ground/water creates more complex temperature gradients. These don't simple shift the figure one way or the other, they create more complex situations, like where the light gets bent around the curve creating an image that is highly compressed at the bottom, but not really at the top. A classic example here is the view of Toronto from Fort Niagra:

Here's the expected view with no refraction and flat earth:

But what we are seeing here is a compression of things close to the horizon. Here I've simulated this on the left, transitioning to the "correct" view on the right:

Or with the full image:

So it would be misleading to try to put a range on the value used to adjust r (7/6), especially if it's used for simple lights, like lasers and lighthouses. Frequently these are visible in the highly compressed portion of the image near the horizon, where there are no discernable details of the scene. Sometimes that compressed portion is so thin and essentially featureless that you'd have a hard time knowing it was there.

So I'd rather try to draw attention to this compression than place some arbitrary limit on how far away you can see a laser light.

 

[tommyt]

Hi Mike,

Thanks so much for this post.

I have a question though that I haven't found an answer for and hoping you can help.

Your illustration and calculations gives me the hidden altitude over a target, over the horizon a given distance and eye height away from that target. That's all fine and easy.

What I can't figure out is the FORMULA for the new hidden altitude to the target if there is a object between eye level and the horizon essentially creating a new horizon.

For example. Let's say my eye level is at 2m. My target is 50km away over a perfectly flat horizon. My visible altitude starts at 158.58m over the target. Everything below that is hidden and not visible.

NOW!!! Let's say there is a 10m tall hill 500m away from my eye level. I can not move. All other constants are the same as above. The hill creates the new horizon 8m over eye level. (10m-2m=8m) What is the NEW visible altitude over the target 50km away?

What formula can I use to figure this?

Thanks and kind regards,
Tom

 

[Mick West]

What I can't figure out is the FORMULA for the new hidden altitude to the target if there is a object between eye level and the horizon essentially creating a new horizon.

See:
https://www.metabunk.org/earth-curve-calculator-for-obstructions.t10406/

 

[Colin Parkinson]

This is something I heard on Godless Engineer and also used with out thinking about it in my own calculations. The observer height should be in feet or meters above the mean sea level. When I did observations on lake Simcoe I used my elevation as my height out of habit. But realized that the curve calculator just had height. The elevation above sea level will have a significant change in the radius used for the calcs. Just a suggestion.
Source: https://youtu.be/R0jR7Cieqr0
in this video taken at salt lake the elevation of 4200 feet makes a big difference to the calcs.

 

[Rory]

Height above the water of the lake will suffice. It's true that you're slightly further away from the centre of the Earth, and that your radius will likely vary from the mean, but it doesn't make a significant difference.

You can vary radius if you click the 'advanced' box, and you can find the radius for your latitude easily enough.

Most of the time, though, using the exact radius only varies the result by a matter of inches.

 

[Rory]

In this video taken at salt lake the elevation of 4200 feet makes a big difference to the calcs.

I was going to look at that in the video, but the video posted seems to be a collection of a great many different observations, experiments, and ideas.

Is that the right video? Or is the observation you referred to at a specific point?

 

[Rory]

I like how the "standard refraction" comes first now, and is highlighted. And the "no refraction" has been termed "geometric".

I've noticed a few people have recently posted screenshots of the calculator where the order is still geometric -> refracted, such as here:

Source: https://www.youtube.com/watch?time_continue=891&v=f7YUYSEYMmo

I wonder why that is?

 

[Mick West]

I wonder why that is?

They are just using old screenshots from old Dr. John videos.

I've added a new note, right in the middle so it will show up in screenshots

This isn't any new information, it's emphasizing something pointed out and addressed in 1870 by Alfred R. Wallace with his Bedford Level experiment. I'm trying to get the FE community to try something other than close-to-water line of sight experiments with bright lights.

Rise up, rise above refraction!

 

[Mathias]

...I'm trying to get the FE community to try something other than close-to-water line of sight experiments with bright lights...

Good luck with that , I'm pretty sure they will do all they can to only observe through the part of the atmosphere that is the most unpredictable.

But with that said I do have good experience with observing from 3-4m in the winter months where the water/air temperature often is about 2-4°C here where I live.

Photo high resolution: https://flic.kr/p/2fP5whP

 

[Mick West]

Then the law of sines to get the dip angle of the base

BaseDip = d/r + Asin((r+h)*Sin(d/r)/EyeToBase) - PI/2

This wasn't working when the target was closer than the horizon as the sine of the intermediate angle L is ambiguous as to if it's greater than or less than 90° (sin(89) == sin(91)). I've fixed this.

When closer than the horizon the "AngularHidden" number is actually the angular size of the object the has the horizon behind it.

I've fixed the diagram and code snipped above. Note this did not change anything when something was actually hidden, which is the main case we are interested in.

 

[Rory]

I just saw a flat earther commenting that they were doing some observations over a bay from 20 feet above sea level, and that they were "sure the metabunk calculator 'accounts for atmospheric refraction'" but that it states that "it doesn't work over bodies of water."

The text on the calculator page currently says: "Not accurate for observations over water very close to the horizon (unless the temperature and vertical temperature gradient are accurate)."

I feel a bit confused by both the above. First, the flat earther who thinks the calculator itself states that it doesn't work over water, garnered from reading the note; and second, the words "very close to the horizon".

Isn't the point that it isn't as accurate when the observer is close - low down; within say 10 or 15 feet - to the body of water?

 

[Rory]

The above point is being discussed right now on a YouTube panel debate. From listening to them, it seems that it would really benefit to define "close" more specifically. One person thinks "close" means six inches but not six feet; another thinks six feet is "close". And there may be others who think twenty feet is "close".