Gimbal Blender Simulation with Clouds

[Edward Current]

I'm getting the first results from my Blender simulation. Here is a side-by-side comparison from the beginning of the video:

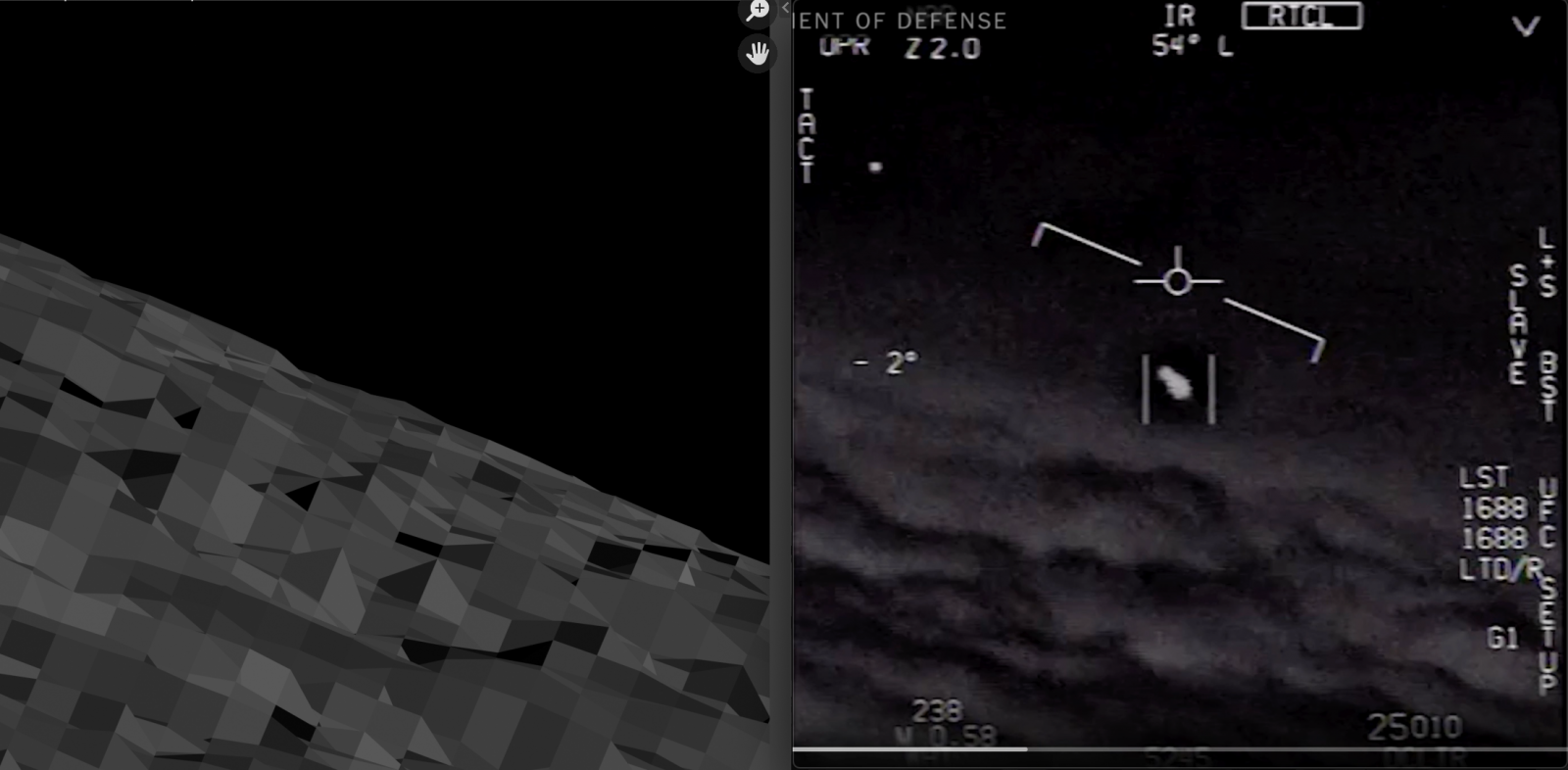
In order to get the "clouds" properly in the frame, I raised them by 2,500 feet; initially I had them topping out at 6,500 feet, and now they top out at 9,000. Additionally I lowered the camera's pitch from –2.0° to –2.3°. Raising the clouds higher without changing the camera pitch, or increasing the camera's downward pitch without changing the cloud altitude, produce similar results, although if we accept the camera pitch as being somewhere between –1.5° and –2.5°, the maximum height of the clouds is constrained (I'll work out all of the numbers).

Unfortunately Blender won't let me do a FOV smaller than .367°, so eventually I'll mask out the edges to get a .35° or .25° FOV (see above).

Keeping in mind that this setup represents only the above chosen set of parameters, I measured the distance to the "clouds" in the frame. Near the bottom of the picture, they are 9.4 NM away 94 NM away. Those at the crest of the horizon are on the order of 15 NM 150 NM away.

Therefore, according to this analysis, the Gimbal object is inside of 9 NM (unless it's moving fast enough to overcome the parallax effect). I'm surprised by this result — I thought it would be farther — but there are still some uncertainties. I'll be able to place tighter constraints on the distance when I add the object and begin replicating the motion of the clouds.

 

[TheCholla]

@Edward Current , that's great you're attempting this. I'm surprised by your number, how can a plane looking down 2deg below the horizon can see 3000m (9000ft) clouds that are only 10Nm further? I was visualizing something like this, but maybe it's dead wrong? Do you know what explains the discrepancy with your result ?

It's simply using the F-18 altitude, angle below the horizon, to see how a cloud layer at a certain altitude would position compared to the FOV. Here it's for a cloud layer at 3000m.

 

[Edward Current]

Do you know what explains the discrepancy with your result ?

Yes, and man, you nailed me there. I was off by an order of magnitude. I saw 570000 (feet) on the calculator and read it to Siri as 57,000.

The distance to the clouds at the bottom of the picture is 94 NM. I hope that works better for you. It's certainly closer to the 120 NM ballpark estimate that I made in that other thread, based on the angular size of the cloud features.

 

[TheCholla]

Ok that makes more sense! Looking forward to the rest of your reconstruction with the object.

 

[jarlrmai]

Yes, and man, you nailed me there. I was off by an order of magnitude. I saw 570000 (feet) on the calculator and read it to Siri as 57,000.

The distance to the clouds at the bottom of the picture is 94 NM. I hope that works better for you. It's certainly closer to the 120 NM ballpark estimate that I made in that other thread, based on the angular size of the cloud features.

Do your cloud layers follow the Earth's curve? It makes a big difference.

Also how confident can we be about the size of the cloud features and the distance surely we run into similar issues with them that we do with the size/distance of the object itself?

This was he main issue I had with my Blender reconstructions.

 

[Edward Current]

Do your cloud layers follow the Earth's curve?

Yes (there's also a planet under the clouds).

Also how confident can we be about the size of the cloud features and the distance surely we run into similar issues with them that we do with the size/distance of the object itself?

I'm not confident at all about the size of the cloud features; that was just a back-of-the-napkin estimate. But that number has nothing to do with the new measurement from the simulation. The measured distance to the clouds is just a matter of where they show up in the simulated picture. (I went into edit mode, selected some vertices at the bottom of the picture, then switched to overhead orthographic view and measured the distance to the highlighted vertices.)

The accuracy of the simulation measurement assumes that we know the diameter of the Earth, that the clouds roughly follow the Earth's curvature, and that Blender's field of view is what it says it is. What we aren't certain of is the ATFLIR's exact FOV spec. Changing the altitude of the clouds vs. the precise downward camera pitch makes a difference, but it isn't huge. I'll figure out these ranges. Given my face-plant blunder just above, I will definitely want people to check my simulation for other dumb mistakes.

 

[Edward Current]

Why the ATFLIR angle numbers do not change smoothly: While setting keyframes for the yaw angle in my Gimbal recreation, I noticed that new onscreen angle numbers appear only on frame numbers that are multiples of 6 (with an offset). This means, I think without any doubt, that the ATFLIR system updates this reading only 5 times per second. That explains why, when entering the data by the exact frame number, the movement of the camera appears to be herky-jerky and needs to be smoothed. (One Twitter user claims this is objective evidence that the Gimbal object was moving erratically or even in small circles.)

This is only verifiable in the version downloaded from the DOD. Other versions that I had on my hard drive (notably, the one from the New York Times which I had screen-captured) have repeated and skipped frames.

 

[Mick West]

Why the ATFLIR angle numbers do not change smoothly: While setting keyframes for the yaw angle in my Gimbal recreation, I noticed that new onscreen angle numbers appear only on frame numbers that are multiples of 6 (with an offset). This means, I think without any doubt, that the ATFLIR system updates this reading only 5 times per second. That explains why, when entering the data by the exact frame number, the movement of the camera appears to be herky-jerky and needs to be smoothed. (One Twitter user claims this is objective evidence that the Gimbal object was moving erratically or even in small circles.)

This is only verifiable in the version downloaded from the DOD. Other versions that I had on my hard drive (notably, the one from the New York Times which I had screen-captured) have repeated and skipped frames.

Notably the numbers in GoFast exhibit the same behavior. I only checked for 50° to 56°, but they are, as you say, multiples of 6

50° - 21:06 (times are in seconds:frames at 30 fps)
51° - 23:06 (+2:00)
52° - 24:18 (+1:12)
53° - 26:06 (+1:18)
54° - 27:18 (+1:12)
55° - 29:06 (+1:18)
56° - 30:12 (+1:06)

Yet there's no evidence of this jerky behavior in the smooth tracking across the ocean background.

So they absolutely have to be smoothed.

 

[Mendel]

we know the diameter of the Earth,

Are you using a 7/6r compensation for refraction because of the near-horizontal view?

 

[Edward Current]

Are you using a 7/6r compensation for refraction because of the near-horizontal view?

I am not. The refraction is due to temperature and pressure variance by altitude, right? That could get tricky. Part of the sightline passes through a thermal inversion. We can assume this because the clouds appear to be stratocumulus, whose tops are kept at a very consistent altitude by a stable (inverted) layer of air above, where there's no convection. But, the depth and gradient of the inversion layer is unknown.

Edit: I did some experiments. If I scale up the Earth and the cloud layer by 17%, the camera is looking at clouds only; but I can restore the appearance of the clouds in the video if I lower them back down close to their original altitude of 6,500 feet. Having done that, the distance is 103 NM to the nearest clouds in the picture, rather than 94 NM. I'm happy to go with 7/6 x R if you think that's appropriate.

 

[Mick West]

I am not. The refraction is due to temperature and pressure variance by altitude, right? That could get tricky.

Very tricky. The 7/6 rule of thumb is for sightings more-or-less at sea level, and I think this will change when looking down from 25000 feet.

Here's a 20 foot high target 81 miles away 11,443 feet altitude, viewed from 25,000 feet, 0.35° vertical FOV, looking down 2.4°, standard refraction, and no refraction.

So adding standard refraction (assuming my simulator is working, which I think it is based on previous accurate replications) is raising the 80 mile distant target by about 1/4 of the 0.35 FOV, or about 0.0875°

 

[Mick West]

Regarding refraction, using LWIR (3700 to 5000 nm, the range used by ATFLIR) makes no significant difference, just a very slight lowering of the target.

 

[Mendel]

Very tricky. The 7/6 rule of thumb is for sightings more-or-less at sea level, and I think this will change when looking down from 25000 feet.

I felt that the viewing angle of -2⁰ is close enough to horizontal for this to be similar (i.e. not "looking down").
The optical density gradient results from the adiabatic temperature/pressure curve, so refraction is going to be present in some form, only its strength may depend on altitude?

 

[jplaza]

As a first approximation, I would leave out refraction. Find a first scenario, worry later about refraction and see how much it changes the result.

 

[Mendel]

As a first approximation, I would leave out refraction. Find a first scenario, worry later about refraction and see how much it changes the result.

Mick just posted that the effect size is ~1/4 of the screen height.

 

[Mendel]

So adding standard refraction (assuming my simulator is working, which I think it is based on previous accurate replications) is raising the 80 mile distant target by about 1/4 of the 0.35 FOV, or about 0.0875°

422.000×sin(0,0875⁰)=644 ft

Metabunk curve calculator has the difference between geometric and refracted drop as 4268-3658=610 ft at 7/6r.

I'd say 7/6r works out well enough, and is easy to implement in the model.

 

[Edward Current]

I'd say 7/6r works out well enough, and is easy to implement in the model.

I made a valiant attempt to simulate atmospheric refraction, by creating layers of atmosphere and giving each layer its own index of refraction, found with various online calculators. Blender can do amazing things with refraction (see below, an exercise I did when learning the program), but it just couldn't handle the precision required — the IORs for air at various temperatures and pressures start off with 1.000..., to say nothing of the distances and angles of incidence involved.

So, 7/6R it is.

 

[Edward Current]

Here is an update on my simulation. I had a very hard time trying to find a flight path that could replicate the cloud motion closely. Even with only 2 bezier points (the beginning and end), the combination of the flight-path curvature and the very steady camera panning produced surprisingly chaotic results. In adjusting the bezier points there are 5 independent variables, and it goes up by 3 for each bezier point added — and it gets increasingly chaotic. It could drive a person mad. I managed to get a pretty good approximation, especially for the first half of the video, with 3 bezier points.

I got much better results once I decoupled the z-rotation of the aircraft+camera from the flight path, and animated the rotation separately. I realized that in the real world, they aren't exactly coupled. Any torquing moment on the aircraft is absorbed by the ATFLIR system. A fraction of a degree of crabbing and whatnot, and the camera would be seeing a different field of clouds, were it not for the system.

In my simulation so far, the aircraft+camera z-rotation deviates the most from the flight path around frame 780 — 1.8°. The flight path could be improved, but I don't know if the aircraft being a couple hundred feet to the left or right at any given point will make a big difference in the end. (Someone on Twitter suggested that finding the best flight path could be done with machine learning.)

So here is the cloud simulation so far. The next step is to put in a UFO, and find some solutions for its trajectory.

 

[Mick West]

So here is the cloud simulation so far.

Something that leaps out to me here is that your simulation seems to have a lot more parallax within the clouds - i.e the distant clouds move more quickly right (in the direction of the plane's travel) than the closer clouds. Could your clouds be too close? Or is there something else that explains why it seems that way?

 

[Edward Current]

Something that leaps out to me here is that your simulation seems to have a lot more parallax within the clouds - i.e the distant clouds move more quickly right (in the direction of the plane's travel) than the closer clouds. Could your clouds be too close? Or is there something else that explains why it seems that way?

I noticed that, too. It's particularly noticeable in the most distant clouds, like the little peak at 0:17, which is 175 NM away. When that peak is midscreen, the round cloud in the lower-left corner is 106 NM. Overhead view with those regions selected:

In the original, I don't think we see those most distant clouds; the range of depth seems much more limited, with anything in the distance dark and blurry. Perhaps I have overdone the variation of the cloud heights. Another factor is that my clouds are reflecting sunlight from a low angle, simulating the look of the white-hot segment — and that could be over-illuminating the distant clouds, which in reality are emitting IR rather than reflecting sunlight. There might be a way I could map their emission level to altitude or surface angle, but I'm still a Blender novice.

 

[Edward Current]

Gimbal Simulation Preliminary Findings

In my Blender simulation, I dialed up 4 scenarios (out of infinitely many) for the Gimbal UAP. Scenario #4 is the most compelling. But first, a review of the setup.

Clouds: Altitude topping out at 7,000 feet. Radius of curvature was increased by 17% to compensate for atmospheric refraction. Under these parameters, the clouds seen by the ATFLIR start at a distance of 106 NM, the most distant being 175 NM (not visible in the original video). Since wind direction/speed is unknown, I had to assume they weren't moving. This may introduce an error.

F-18 aircraft: Flying at 350 knots (591 feet per second) at 25,000 feet. Turn radius starts at approx. 3.5 NM, and reaches a minimum (near end of video) of 2.4 NM. At the end of the simulation, the aircraft has turned to the left by about 64°.

ATFLIR camera: Looking down at a constant 2.22°. Field of view is 0.35° left-to-right. (Measuring 0.35° on the diagonal, which makes the left-to-right FOV 0.25°, did not work. So that hypothesis seems to have been falsified.) The roll of the camera was constrained to the F-18's bank angle, which was animated from an earlier plot. Where necessary for smoothing, I shifted the camera's yaw-angle keyframes by 1 or 2 frames in recognition of the coarse-graining of the onscreen data.

Methodology: From the original video, I identified the frame numbers where the FOV had turned over and new clouds were seen. That was not an exact science, so I repeated the process several times. Toward the end of the simulation, when the cloud movement slows, I counted half-fields. (Since the camera is rolled, more than 0.35° of clouds are seen at any time; I compensated for this.) I then animated a marker to sweep across the clouds with these keyframes, and used auto-bezier to smooth. Finally, the rotation angle of the aircraft/camera was animated to coincide with the marker.

For these 4 scenarios, I favored increasingly tail-on views of the object, on the assumption that we're seeing an engine(s) that increases in brightness — but there are many other solutions.

UAP Scenario #1: Minimal movement, at sightline's pivot point
The object starts at a distance of 22.5 NM, speed ~67 knots, banking slowly to its left. The F-18 is chasing the object and catching up with it. The object's altitude starts at 19,700 feet and climbs to 20,250 feet. At the end, the F-18 is almost on line with the object's trajectory.

UAP Scenario #2: Faster & more distant
Starting distance 66.4 NM, speed ~475 knots, banking slowly to its right (still moving to the left from the F-18's view, but less so over time). It is receding from the F-18. Altitude starts at 9,400 feet and descends to 9,050 feet. This is a surprising result, and is another nail in the coffin of the Atlas hypothesis. (Unless it's a SRB that's still firing as it descends — do they do that?) An object at this distance needs to be fast to stay in the FOV, but slightly less so if moving perpendicular to the line of sight.

UAP Scenario #3: Constant altitude (13,500 feet)
Starting distance 46.6 NM, speed ~373 knots, banking slowly to its right. It is receding from the F-18. Much slower (~200 knots) if moving perpendicular to line of sight.

UAP Scenario #4: Moving like a small jet
This is close to Scenario #1, but with a faster receding speed: Starting distance 22.5 NM, speed ~300 knots, banking to its left with a turn radius of 10 NM.

One interesting thing about this scenario is that like #3, it is also constant altitude — 19,700 feet. Which is where Scenario #1 starts. But, this object is moving fast enough to escape the approach of the F-18 (which tends to make objects at lower altitudes move down in view), and this, remarkably, keeps the top of the clouds in the center of the FOV.

The other interesting thing about this scenario is that the F-18 is looking straight up the object's backside, so to speak, even as both turn.

So here we have a scenario that's quite unusual: An F-18's ATFLIR locks onto an object that appears to be moving steadily against the clouds for over 30 seconds, and during that interval, in the picture, the object is maintaining both its height relative to the clouds and its IR brightness as the F-18 continues to look from directly behind. Add to that the "it's rotating" business at the end, and you have the most famous UFO video ever.

 

[Mick West]

UAP Scenario #4: Moving like a small jet
This is close to Scenario #1, but with a faster receding speed: Starting distance 22.5 NM, speed ~300 knots, banking to its left with a turn radius of 10 NM.

This is the one that makes the most sense to me. Constant altitude is simplest. Moving to the left seems required as it keeps moving relative to the clouds long after parallax could account for it. Distance is sufficiently far that they would not easily eyeball a small jet. Speed seems consistent with a small jet.

 

[Edward Current]

This is the one that makes the most sense to me. Constant altitude is simplest. Moving to the left seems required as it keeps moving relative to the clouds long after parallax could account for it. Distance is sufficiently far that they would not easily eyeball a small jet. Speed seems consistent with a small jet.

Given that the more distant scenarios bank to the right (which is very counterintuitive — see below), I suspect there's a unique solution that's both constant-altitude and straight-line. If unlike Scenario #4 it starts at a small angle of incidence that gets even smaller, accounting for the increase in apparent size of the glare, that would be the ultimate brass ring.

What would be typical flight characteristics of a small jet going or coming from, say, the Bahamas? Would the altitude be on one of the 1000s?

 

[Mick West]

What would be typical flight characteristics of a small jet going or coming from, say, the Bahamas? Would the altitude be on one of the 1000s?

Jets generally stick to a multiple of 1000 feet. In controlled airspace it's whatever ATC tells you. Otherwise there's some general rules based on altitude and heading.

Article:

(1) When operating below 18,000 feet MSL and -

(i) On a magnetic course of zero degrees through 179 degrees, any odd thousand foot MSL altitude (such as 3,000, 5,000, or 7,000); or

(ii) On a magnetic course of 180 degrees through 359 degrees, any even thousand foot MSL altitude (such as 2,000, 4,000, or 6,000).

(2) When operating at or above 18,000 feet MSL but below flight level 290, and -

(i) On a magnetic course of zero degrees through 179 degrees, any odd flight level (such as 190, 210, or 230); or

(ii) On a magnetic course of 180 degrees through 359 degrees, any even flight level (such as 180, 200, or 220).

(3) When operating at flight level 290 and above in non-RVSM airspace, and -

(i) On a magnetic course of zero degrees through 179 degrees, any flight level, at 4,000-foot intervals, beginning at and including flight level 290 (such as flight level 290, 330, or 370); or

(ii) On a magnetic course of 180 degrees through 359 degrees, any flight level, at 4,000-foot intervals, beginning at and including flight level 310 (such as flight level 310, 350, or 390).

(4) When operating at flight level 290 and above in airspace designated as Reduced Vertical Separation Minimum (RVSM) airspace and -

(i) On a magnetic course of zero degrees through 179 degrees, any odd flight level, at 2,000-foot intervals beginning at and including flight level 290 (such as flight level 290, 310, 330, 350, 370, 390, 410); or

(ii) On a magnetic course of 180 degrees through 359 degrees, any even flight level, at 2000-foot intervals beginning at and including flight level 300 (such as 300, 320, 340, 360, 380, 400).

Source: https://www.law.cornell.edu/cfr/text/14/91.179

"altitude" is absolute altitude - assuming you've corrected your altimeter for local pressure.

"flight level" is a nominal altitude with altimeter ignoring local pressure and set to 29.92 inches Hg - that means that it's not exactly on the 1,000 foot multiple when at or above 18,000 feet, but it is when below.

I think most airspace is RVSM now (after 2005).

 

[Edward Current]

Scenario #5: Straight line, constant altitude
I believe I found the solution I am looking for. The starting distance is 29.4 NM, speed ~375 knots, altitude 19,200 feet. A straight-line trajectory at constant altitude is the simplest case, although it seems a bit fast for that altitude.

As I'd hoped, in this scenario the angle between the sightline and the object's trajectory decreases as it goes: It starts at 15°, and ends at 11° (measured in all 3 dimensions). This could account for the glare in the image getting 20% larger.

The surface of the Earth at distance is not level relative to our xy-plane, so an object maintaining constant altitude is descending in our coordinate system (side view):

This increases its necessary speed — it has to be escaping the F-18 to stay above the clouds in the picture. Eventually this and similar trajectories disappear below the horizon.

 

[Easy Muffin]

I think most airspace is RVSM now (after 2005).

Yes, it's been applied virtually everywhere these days. Some countries use a north/south separation instead to accomodate the predominant direction of their traffic flows, the high North Atlantic airspace can have traffic on published tracks every 1000 ft in the same direction, some might have local regulations etc (traffic in the area of the South China Sea flares incident for example seems to be on odd or even levels on different days - not sure what's up with that).

IFR traffic in North America follows the standard RVSM rules though,
westbound - ... 320, 340, 360, 380, 400, 430, 470, 510 ...
eastbound - ... 310, 330, 350, 370, 390, 410, 450, 490 ...

 

[Mendel]

The starting distance is 29.4 NM, speed ~375 knots, altitude 19,200 feet. A straight-line trajectory at constant altitude is the simplest case, although it seems a bit fast for that altitude.

Well...

F-18 aircraft: Flying at 350 knots (591 feet per second) at 25,000 feet.

The speed of sound is 614 knots at 20,000 feet, so 375 knots is only 0.61 Mach.

Why do you say that it's "a bit fast for that altitude"?

 

[Mendel]

The surface of the Earth at distance is not level relative to our xy-plane, so an object maintaining constant altitude is descending in our coordinate system (side view):

This increases its necessary speed — it has to be escaping the F-18 to stay above the clouds in the picture. Eventually this and similar trajectories disappear below the horizon.

I don't understand the logic here. An object maintaining altitude will appear to move down as it moves away ("escapes") because the planet is curved. For it to maintain altitude and not appear to descend, it needs to maintain its distance = move at the same speed.
You seem to be stating the opposite?

 

[Edward Current]

The speed of sound is 614 knots at 20,000 feet, so 375 knots is only 0.61 Mach.

Why do you say that it's "a bit fast for that altitude"?

I just don't know why a small jet would cruise at that altitude when 30k would be much more efficient.

I don't understand the logic here. An object maintaining altitude will appear to move down as it moves away ("escapes") because the planet is curved. For it to maintain altitude and not appear to descend, it needs to maintain its distance = move at the same speed.
You seem to be stating the opposite?

Past 135 NM, where the tilt of the Earth starts to exceed the camera's 2.22° angle, the object either has to climb to stay in line with the clouds, or be receding (and moving transversely) by fantastic speeds. Closer than that, if maintaining its altitude, it needs to recede fast enough to overcome the smaller tilt of the Earth, with a recession velocity of zero at the local "flat Earth" limit. If they're co-moving at 50 NM, for example, a constant-altitude object will appear to descend because it is moving along a tilt and the F-18 (essentially) isn't. I'm working on this today and will have diagrams.

 

[Mick West]

If they're co-moving at 50 NM, for example, a constant-altitude object will appear to descend because it is moving along a tilt and the F-18 (essentially) isn't. I'm working on this today and will have diagrams.

I might be misunderstanding what you mean here. Surely if the distance between two objects at fixed altitudes remains the same, no matter how far apart they are, then the slant angle between them will also be the same? So are you just talking about a portion of the video, like in the early bank, when the closing velocity will be smaller?

 

[Edward Current]

I might be misunderstanding what you mean here. Surely if the distance between two objects at fixed altitudes remains the same, no matter how far apart they are, then the slant angle between them will also be the same? So are you just talking about a portion of the video, like in the early bank, when the closing velocity will be smaller?

Yes, I think the generalization I made neglects local curvature. If you are walking on flat ground and a friend ahead of you is walking on a downslope, your friend will appear to descend and may disappear from view. But if you are both walking along a large ball, your friend will never change level.

I'm trying to come up with a plot of final recession velocity (at the end of the video, because it changes as noted) vs distance, in order to find two lines: one connecting constant-altitude solutions, and one connecting straight-line solutions. In the simulation, counterintuitively, all solutions closer than a certain distance bank to the F-18's left — whether coming or going — and those farther away bank to the right. It may be that the line for constant-altitude solutions is simply zero recession velocity at all distances.

 

[Edward Current]

Further Analysis of the Gimbal Simulation in Blender

My Blender simulation with clouds puts significant constraints on theories about the movement of the Gimbal object. The results apply directly to an idealized case where the clouds are stratocumulus topping out at 6,500 feet, with negligible movement due to wind. However, I expect that if the clouds were slightly lower or higher (to the extent allowed by the camera tilt), or if they were moving at a wind rate typical of these altitudes, or if the F-18's flight-path curve were slightly different, those constraints would be shifted — but not eliminated.

Having run exploratory scenarios #1-4, plus others not published, I found there is a family of trajectories where the object has a constant altitude (as measured normal to the Earth's surface), and also where the object goes in a straight line (does not bank left or right). Here's how all ordinary-type scenarios lay out on a plot of final recession velocity vs. distance:

Scenario #5 is the unique solution where the object is moving straight and level: 377 knots, 18,770 feet. A couple of counterintuitive results: First, how the constant-altitude line takes off with distances past 60 NM — this is due to the object's having to move at increasingly transverse speeds with distance due to the sweep of the camera. Also, that a distant, approaching object banks to the F-18's right. But it never actually moves to our right; it is just turning more toward the F-18 as the camera sweep slows over the course of the video.

Here are the constant-altitude scenarios as seen from overhead:

And, the straight-line scenarios:

I suppose that Scenario #13, if combined with fairly strong winds at 6,500 feet, could allow for a "motionless" object at 26.6 NM (but it rises at 19 feet per second). I did not find any scenarios where the object can come to a stop and change direction, even as seen overhead — but there exist more complex solutions that I haven't looked into. I assume there are some with changing velocities (including vertical and angular), but I think multiple parameters would have to change in coordination just to resemble, from the camera's view, much simpler scenarios.

Here are all of the numbers for the scenarios plotted:

The angles of incidence are with the trailing end of the object. Assuming we're looking up the throat of an engine or engines, some scenarios (5, 10, 11) are consistent with the observed increase in the size of the glare; others are not. With the exception of initial distance, which is a ground measurement, all distances/velocities/angles are measured in 3 dimensions.

I've uploaded the Blender file here. Next I will re-output Scenario #5, add some effects to more closely recreate the original video, and then make a presentation for YouTube.

 

[Mendel]

Scenario #5 is the unique solution where the object is moving straight and level: 377 knots, 18,770 feet.

This obviously depends on your assumption that the observing aircraft moves at 350 knots.

F-18 aircraft: Flying at 350 knots (591 feet per second) at 25,000 feet.

How did you arrive at that?

Your scenario 5 puts the UAP at ~30 nm; what does that tell us about its size?

 

[Edward Current]

This obviously depends on your assumption that the observing aircraft moves at 350 knots.

Yes. If it's faster or slower, the results will be shifted. I'd be happy to try a different speed or speeds — I haven't done so yet.

How did you arrive at that?

From way back here. Maybe that estimate has been updated?

Your scenario 5 puts the UAP at ~30 nm; what does that tell us about its size?

At the beginning of the video in white-hot mode, the glare covers a region about 70 feet across, and about 40 feet high (along the z-axis).

 

[Mendel]

From way back here. Maybe that estimate has been updated?

The display shows a calibrated air speed of 241-242 knots, and Mick converted that to 350 knots true air speed.

Thank you!

 

[jarlrmai]

The display shows a calibrated air speed of 241-242 knots, and Mick converted that to 350 knots true air speed.

Thank you!

For a blender sim any speed used would need to be ground calibrated.

 

[Mendel]

For a blender sim any speed used would need to be ground calibrated.

Why? The wind speed affects everything equally ( Navy aircraft, clouds, UAP), so it cancels out.

 

[markus]

Why? The wind speed affects everything equally ( Navy aircraft, clouds, UAP), so it cancels out.

There can be a significant difference between wind speeds as a function of altitude. For example, the quoted "120 knots" wind would be considered a category 4 hurricane near the ground. I can't find it anymore but someone had posted a website with the measured winds at various altitudes in the day when this event supposedly take place; that'd be good to try and estimate that wind shear.

Also, the calibrated airspeed -> true airspeed conversion is a notable point of uncertainty since the conversion depends on the local air density, which is unknown. We know we're at a barometric altitude of 25k feet which is straightforward enough to convert to pressure but finding the density further requires knowing the temperature.

@Edward Current I'm sorry if you posted this before, but how do you know the altitude of the cloud layer? Is it an assumption? If so it seems a very important one.

 

[Mick West]

For a blender sim any speed used would need to be ground calibrated.

Why? The wind speed affects everything equally ( Navy aircraft, clouds, UAP), so it cancels out.

Since (as markus said), the wind can be different at different altitudes (and even with distance, although less so), just using an assumed wind of 0 is an oversimplification. However you can assume it does not change for the jet (i.e. a local wind speed of 0) then the local wind for the object and the clouds is just a component of their velocity. It's the relative speeds which are important. You can translate that to a ground speed by adding in an arbitrary jet-local wind velocity, but it won't make a huge difference. The first goal would be see what the possible relative motions are.

However we do have one datapoint of "120 knots out of the West", which is probably the jet-local wind. So you might as well use that.

 

[Mick West]

@Edward Current I'm sorry if you posted this before, but how do you know the altitude of the cloud layer? Is it an assumption? If so it seems a very important one.

There's some discussion here on inferring the cloud altitude from humidity records - but I'm not sure how good a method that is.

https://www.metabunk.org/threads/me...ext=the clouds are where the humidity is 100%

Relative humidity is >100% at 400 hPa, or around 7300 m, but not above. That would make for a cloud layer with a top at ~ 7300 m, a little below the altitude of the fighter.