Z.W. Wolf
Senior Member.
Let's please ignore William Tapley's religious views and his opinion that the flat earth theory is a "psyop" and just concentrate on his mathematical argument. Is it correct?
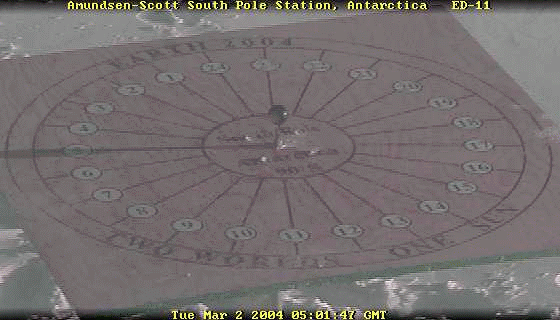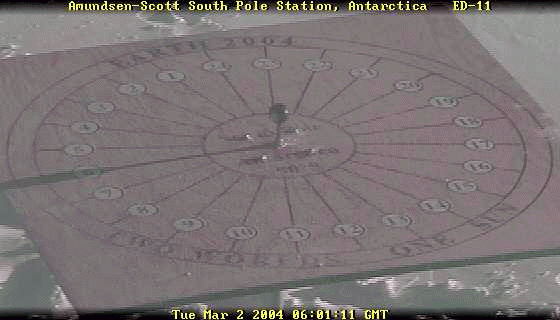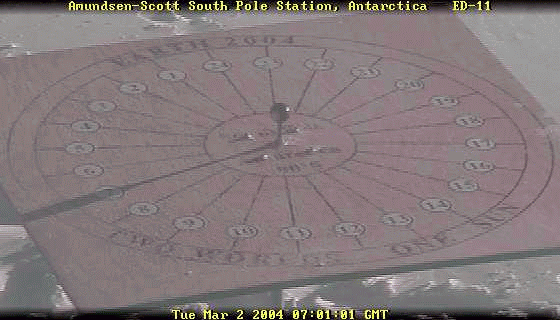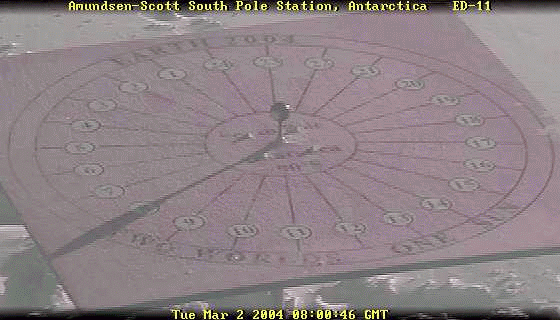
In this video, Tapley is making the argument that on a spherical earth, the shadow cast by a gnomon will increase in length geometrically over equal amounts of time - which is what we observe. On a theoretical flat earth, the shadow cast by a gnomon would increase in length in an arithmetic progression over equal lengths of time - which we do not observe.
Is there anything wrong with his theoretical flat earth model and the equidistant shadows? A flat earther pointed out in the comments, that in Tapley's model, the sun is traversing the earth laterally - in a straight line. But in the flat earth model, while the sun does move in a plane parallel to the earth's surface, it is also following a circular path over the surface.
A question to our mathematicians here. Would that second factor - the circular motion - change or invalidate the argument? Would the shadows cast by a gnomon be equidistant over the course of the day?
In this video, Tapley is making the argument that on a spherical earth, the shadow cast by a gnomon will increase in length geometrically over equal amounts of time - which is what we observe. On a theoretical flat earth, the shadow cast by a gnomon would increase in length in an arithmetic progression over equal lengths of time - which we do not observe.
Is there anything wrong with his theoretical flat earth model and the equidistant shadows? A flat earther pointed out in the comments, that in Tapley's model, the sun is traversing the earth laterally - in a straight line. But in the flat earth model, while the sun does move in a plane parallel to the earth's surface, it is also following a circular path over the surface.
A question to our mathematicians here. Would that second factor - the circular motion - change or invalidate the argument? Would the shadows cast by a gnomon be equidistant over the course of the day?
Last edited: