The idea of AE projection (azimuthal equidistant projection) is that the distances measured from a certain center point are preserved. The "semi-official" FE map is an AE map North Pole as the center point. So on the FE map, the distances measured from the North Pole are the same as on the globe map. In general, all distances measured along meridians are correct on the FE map.
Hardly anything else is correct. The areas of the Northern and Southern Hemispheres are of course the same in reality, but on the FE map the area of the southern "semi puck" is three times the area of the northern one. The FE map magnifies all areas and all distances (except those measured along meridians). Here are some examples of these "stretch factors":
– Earth's surface area: 2.46
– Northern Hemisphere area: 1.23
– Southern Hemisphere area: 3.70
– Length of the Equator: 1.57
– Distance in east-west direction at latitude 60°: 1.04
– Distance in east-west direction at latitude -60°: 5.19
– Distance from Perth to Sydney: 2.52
In Finland the FE map does not have very large distortions. In Helsinki (latitude 60), the east-west distance is only approx. 4% too long, further north even less. Distortions increase in the southern hemisphere. The most dramatic is the situation at the South Pole, where one point stretches into a circle about 125,700 km long.
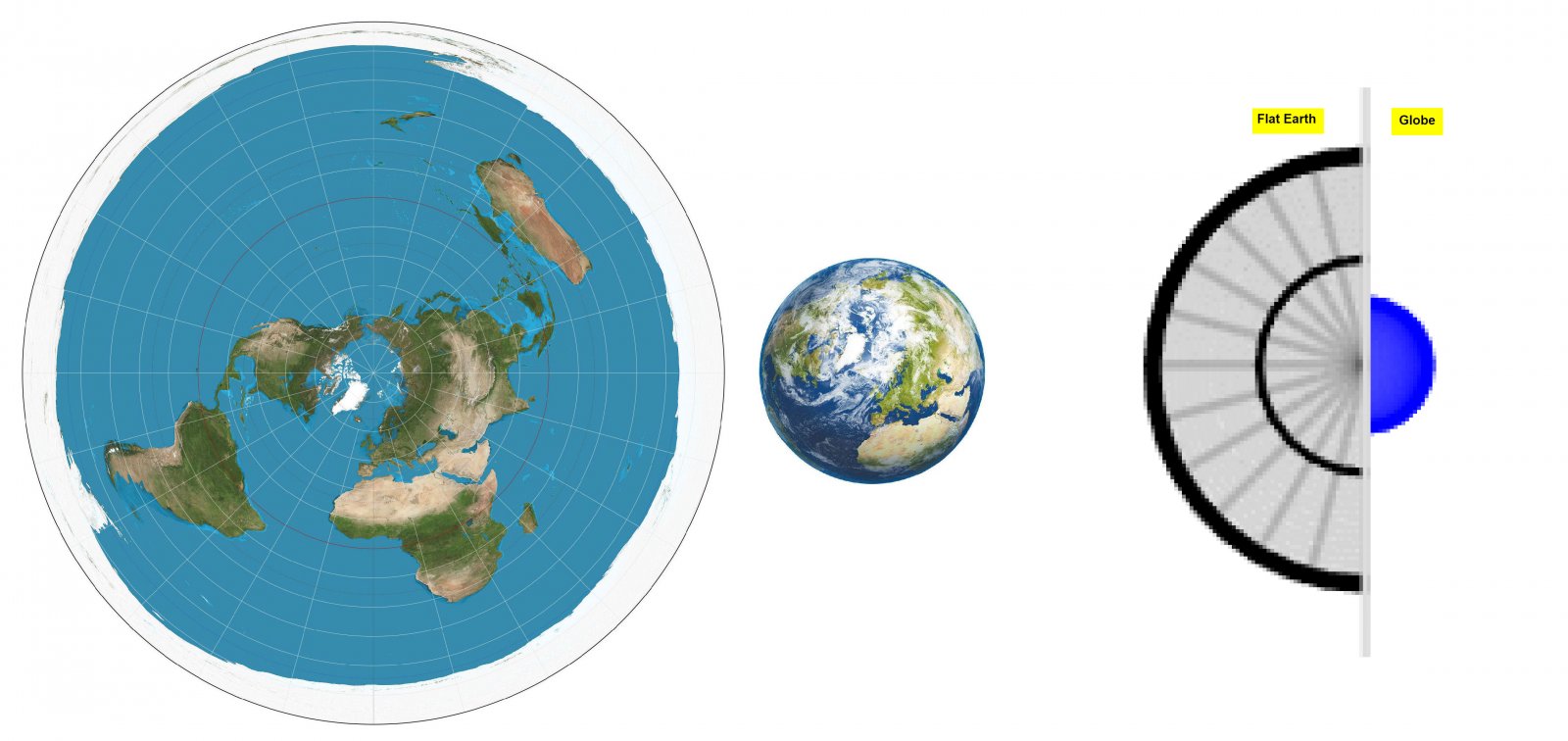
Below left are FE and GE to scale. The diameter of the FE disk is 40000 km, which is also the circumference of the Earth.
Below right is a magnified screen shot from Bislin's calculator. There are flat Earth and Globe side by side seen from a very long distance (70,000 km).
http://walter.bislins.ch/bloge/inde...53-9-9-9-1~0.0343-10-10.00373383-1~86.204-9-4.
The ratio in these comparisons is about the same. So we can conclude that the radius of the flat Earth is 20000 km in Bislin's calculator too.
Another useful calculator made by Bislin is "Creating Flight Plans for Flat Earth",
http://walter.bislins.ch/bloge/index.asp?page=Creating+Flight+Plans+for+Flat+Earth. It is easy to compare FE and GE distances by providing the coordinates of the locations. You get the coordinates e.g. from Google Maps by right-clicking on a place. Then click on the coordinates in the context menu and they will be copied to the clipboard in decimal format.
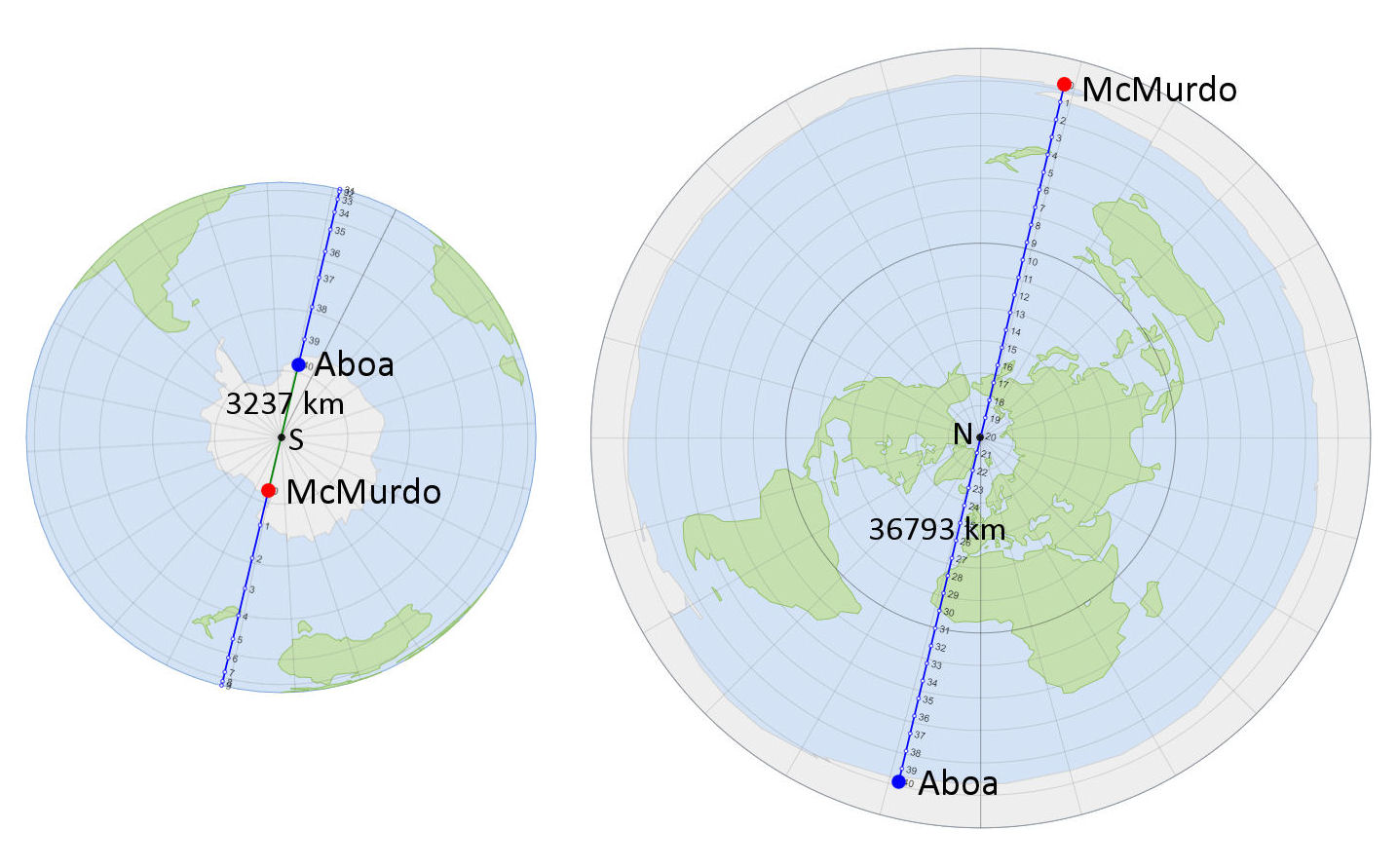
Most flat-earthers admit the existence of research stations located on the coast of Antarctica. Such are Finland's Aboa (-73.048,-13.420) and USA's McMurdo (-77.842,166.688). The direct route between these stations happens to go very precisely through the South Pole.
The image on the left shows the distance between these stations on the globe map (green segment). It is about 3240 km. That's roughly the distance between Detroit, Michigan and Sacramento, California.
On the right is the same journey on FE map. The shortest route between the stations goes through the North Pole. The length of the trip will be approx. 36,790 km.
Yes, the journey from Aboa to McMurdo could be made via the North Pole on the globe also. The length of this trip would also be 36,790 km. This is because the journeys are made along meridians, so both maps give the same values.
As a joint project between Aboa and McMurdo, a research flight from one station to another could be carried out. Leading figures of the FE community would also be included, agreeing to all their demands (research equipment, food, etc.) A stopover could be made at the South Pole in Amundsen Scott station. There could the researchers spend a night watching the midnight sun. (The station does have accommodation for tourists.)
Could this journey show to the flat-earthers which map was followed on the trip? Was the distance 3200 km or 37000 km? Perhaps something could also be deduced from the landscapes below.
If the budget of the research stations is not enough for this public education project, one could ask some of the world's many billionaires as a sponsor. They might be interested in the publicity value of the trip, especially if their own press people were going along. "Flat Earth supporters at the South Pole admiring Midnight Sun" sounds a very attractive tabloid headline.