Question.
Would smashing two Hot Wheel cars together render accurate damage results to show what would happen in an actual car crash?Mar 27, 2021
Can you guess what the challenge is?
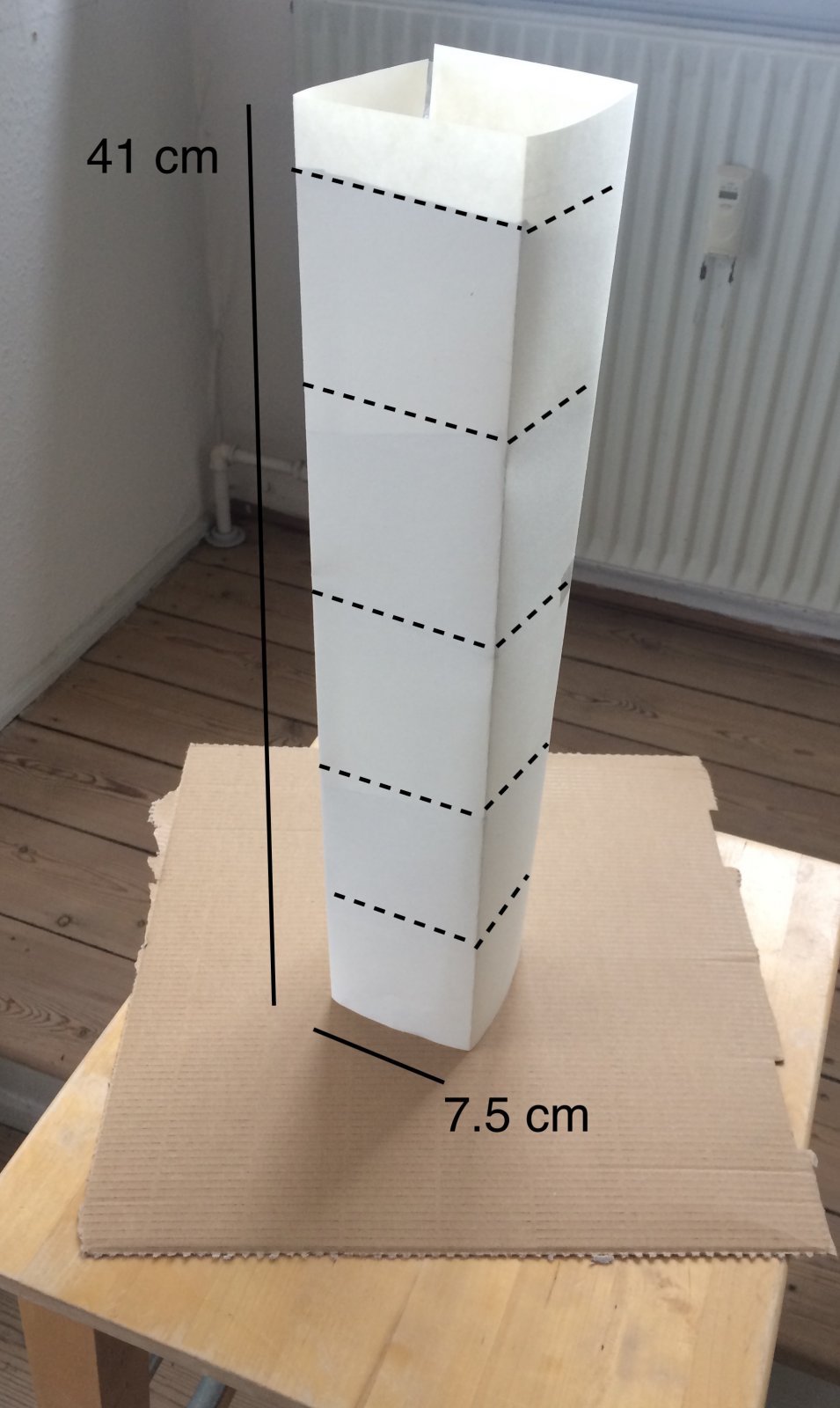
The paper is 30 cm x 41 cm and 90 g/m2.
The cardboard is basically what you get in a pizza box, cut into 7.5cm squares.
Those are AA Duracell batteries. You must put an equal amount of batteries on each floor and the floors must be equally spaced throughout the tower.
My (not rhetorical) question is: in order to use these sorts of materials to meet (something like) Hoffman's challenge, how big would you need the piece of paper & cardboard squares to be? (You can also specify the grade of paper if you like.)
Ideally, you will keep the floor heights and bases [spans] equal, so that the structure is a series of cubes, but I'm willing to hear why that's unreasonable.
If you want more or bigger (or smaller) batteries, just ask!
You can also cut holes in the paper as you choose to weaken the walls.
You can (and probably should) connect the tower to the base (the big piece of cardboard) using as much tape as you like. If you need a bigger or heavier base, that can also be arranged.
The structure must be strong enough in its initial state to let me poke a hole through the paper with the scissors at any point.
The structure must also be strong enough to let me shift the base about 10% of the width of the tower back and forth instantaneously (simulating an earthquake). I think that makes any "wind testing" moot.
The initiating failure must be brought about using the scissors in the ordinary way, cutting the paper, cardboard, or tape as much as you like somewhere in the top 20% of the structure.
This event must cause the whole structure to be destroyed. (It's not good enough that all the floors and batteries end up at the bottom of the tube, which remains stranding.)
The solution using the smallest piece of paper wins.
(Note: you are of course free to ignore this challenge, ridicule it, or meet it as you choose. Once it occurred to me I thought it was too good to keep to myself. I don't think I'm going to be able to meet it with the 30x40cm piece of paper I have. But I'm going to try.)
[The thing I like about this challenge is that it lets us scale up instead of down. That is, if we can't get the 41 cm tower to progressively collapse because gravity doesn't scale well, we can imagine it -- or even build it -- bigger until we reach floor heights where a gravity-driven collapse becomes possible.]
No, it would just be strong enough to support the equavalent vertitcal load. Like I say, it just means that the four connections together could carry the weight of four floors.
What result determines when you stop scaling and that you have all the components correctly modeled?
No, but two paper cars -- actually, one car and one semi truck -- with lead weights for engines (and passengers and cargo)
I'm not sure, exactly. But my first goal is to get the structure to actually collapse. Then I'll see if I'm satisfied with the way it modeled its standing strength.
Then your original statement/assumption you made is incorrect is it not?
Paper correctly scales down to simulate the strength of materials actually used in a car?
Based on your statement above, you should be able to stack four more cardboard floors on one of the floors in your paper model and that supporting floor will collapse correct?
You are merely demonstrating that you still don't understand "scaling in modelling". This is truly hopeless. I am growing more and more convinced that you have made a very conscious, solemn vow with yourself to persist in forever getting scale wrong. "Persisting in being essentially wrong" is, by the way, what I consider to be the best definition of the term "Truther".
How about I don't tap and instead apply a lateral load that slowly increases that models the increasing pull-in of sagging trusses?
Yes, see above: You have made a very conscious, solemn vow with yourself to persist in forever getting scale modelling wrong.
Depending on the weight of paper, yes, roughly. Or that's my thinking at this point, anyway. If there is some other material that better scales the strength of steel down 1000:1, I'm happy to think it through with that too.
If it's so easy and accurate to scale down strength of materials and other characteristics then why do car manufacturers continue to do crash tests with actual cars?
Something like that. But remember that one of the parameters is the load from the batteries (or whatever other unit of weight you propose). So you have to 4x that too. The connections between each floor and the perimeter can be whatever you like, and you of course have to have more weight in the top section than the overload ratio.
I think it depends on what they're testing. Scale models are used in the early stages of the design process, I imagine. And paper or paperboard models can probably be used to investigate various design concepts. (More likely balsawood, I imagine.) Think wind testing, for example. That's often done on small models in materials other than the final product.
They can?
Can you please explain to me the difference between principles of a design and a design concept?
They just have to be strong enough to include some sort of safety factor, but weak enough to be overloaded by the combined weight of the top 20% of floors.
Can you please address post #167?
The design concept (e.g., tube frame) is specific to the product being tested.
What?
Surely you see the difference between carrying the weight of something and actually carrying that thing (in a particular orientation in space)?
I sort of agree with you. I'm reverse-engineering the collapses at 1:1000 scale. Since the buildings collapsed under their own weight, the first order of business is to get the weight distribution roughly right, and then, of course, to have them collapse. I'll go from there.
Surely you can see that saying since one connection can support an entire floor, four of those connections can support four floors is ridiculous right?
Then why is Mick's model not good enough? He got the weight distribution correct didn't he?
I've mentioned this above. Most importantly, Mick's model is 2D. The columns are connected laterally only through the floors and the vertical structure is way too strong in the boards and way too weak at the joints between the boards.
We seem to be seeing this very differently. Suppose every floor in a building weighs 1 ton and it is designed with a safety factor such that a floor fails if loaded with 4 tons. That means we'd need floor connections such that, if there are four of them, they can each carry 1 ton before they
Quite certainly correct
Is it? What makes you think so?
Just to be clear: I mean the joints between lengths of the columns.
Where are the columns and their joints in your paper model?
The columns are represented by the sheet of paper itself. My default model has them as continuous columns. In the WTC, they were of course bolted together in sections. If you think it is necessary, you can introduce weaknesses along the length of the tower by folding the paper, or even by cutting it and joining pieces together.
You say the joints are way too weak. What criteria are you using to make that determination? What measurement are you using to determine a joint/connection is too weak or too strong for the model?
The idea is to have a model that is intuitively strong enough. (When I say "tastes" I just mean my intuitions.) Mick's model just rests column sections on top of each other, relying on friction and gravity to keep them in place. Intuitively, that's not how one would construct a column. One would expect some sort of dowel at the joints.
But you're not, you're on MB talking about doing it. it would probably save alot of time (how many years have you been just talking about this with people who say you're doing it wrong and you saying 'no, i'm not') if you just start doing it.
Your paper model does not have individual columns or joints between them yet you accepted that as ok in spite of your column criteria you just stated.
Yet your model has none of that and you accepted it as ok.
My challenge is to design a structure that stands up under plausible "normal" conditions and collapses after local damage in the top 20% of the structure. If you have a design that works, and joints play a key a role, then I have no objection to them. My hope is that I can make it work without them, though, because it sounds complicated to build.
Without joints/connections?
My model does have spandrels, actually. Solid paper walls are too strong. So I cut out windows at every floor. That leaves four corner columns connected by a strip of paper (the thickness of which I have to get right).
I think I do hold our models to the same standards. And until mine actually collapses I'm not going to claim that mine is better than his. I'm only explaining why Mick's model doesn't satisfy me enough to abandon my own project.
