What figures did you put into the calculator?
The wrong ones, by the looks of things - had my viewer/object radii mixed up.

If I try again, with a slightly different method:
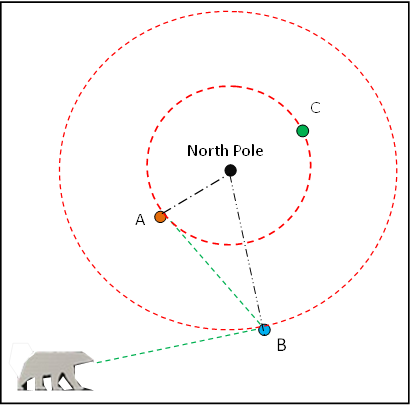
Coordinates for Lake Michigan: 41.905527/-87
Coordinates for Warren Dunes State Park: 41.905527/-86.604366
Coordinates for Willis Tower: 41.8789/-87.6358
Centre of earth to surface of Lake Michigan (577 feet amsl): 20894957.14 feet
Centre of earth to Warren Dunes State Park (@577ft amsl): 20894957.4 feet
Centre of earth to Willis Tower (ditto): 20894990.18 feet
Distance from WDST to WT: 53.07 miles or 280209.6 feet
Viewer elevation above Lake Michigan: 233 feet
Object elevation above Lake Michigan: 18 feet
This time I get 18.7 miles to the horizon and an obscured amount of 735.7 feet.
It's perhaps not all that interesting, and given refraction, etc, still never going to give a precise figure. But seems kind of cool that an equation utilising coordinates could be built into a curve calculator.
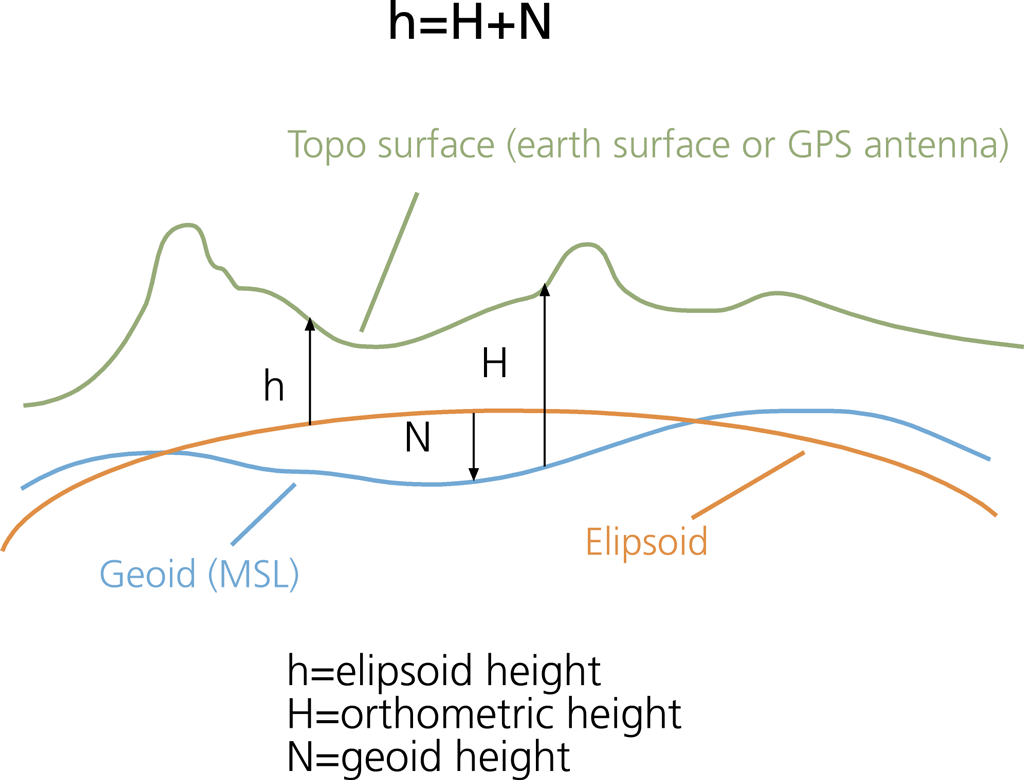
You probably made a mistake. Both places are on almost the same latitude so the effect of the elliptical deformation of the Globe is quite negligible. Using the calculator, I am getting the difference of their Geoid Heights of only 11.4 cm (4.4 inches). I used the coordinates 41.9142562/-86.593821 and 41.8790442/-87.635717
Interesting. I double-checked both our sets of coordinates and both were right. So something's off somewhere.
It does make sense that places at the same latitude should have a similar radius. How about places on the same longitude? If the equatorial radius is 21 miles longer than the meridional radius, would this equate to an average of 890 feet in radius difference for every 50 miles travelled?
As another test, I checked the coordinates for Genoa and Elba - two points in another popular flat earth photograph, this time more north/south - and it returned Elba as having a radius about 1900 feet longer than Genoa.
I guess if viewer elevation is important to the result, such a difference in radius would be too. Or have I gone wrong somewhere again?