Patrick Gonzalez
New Member
So Artemis 1 finally made it to the Moon and it has sent some very gorgeous, high-definition images of not only just the Moon, but also its far side. It's fascinating, but like anything related to spaceflight, flat-earthers find a way to deny everything that disproves their worldview. This image has been doing the rounds on flat Earth Twitter:
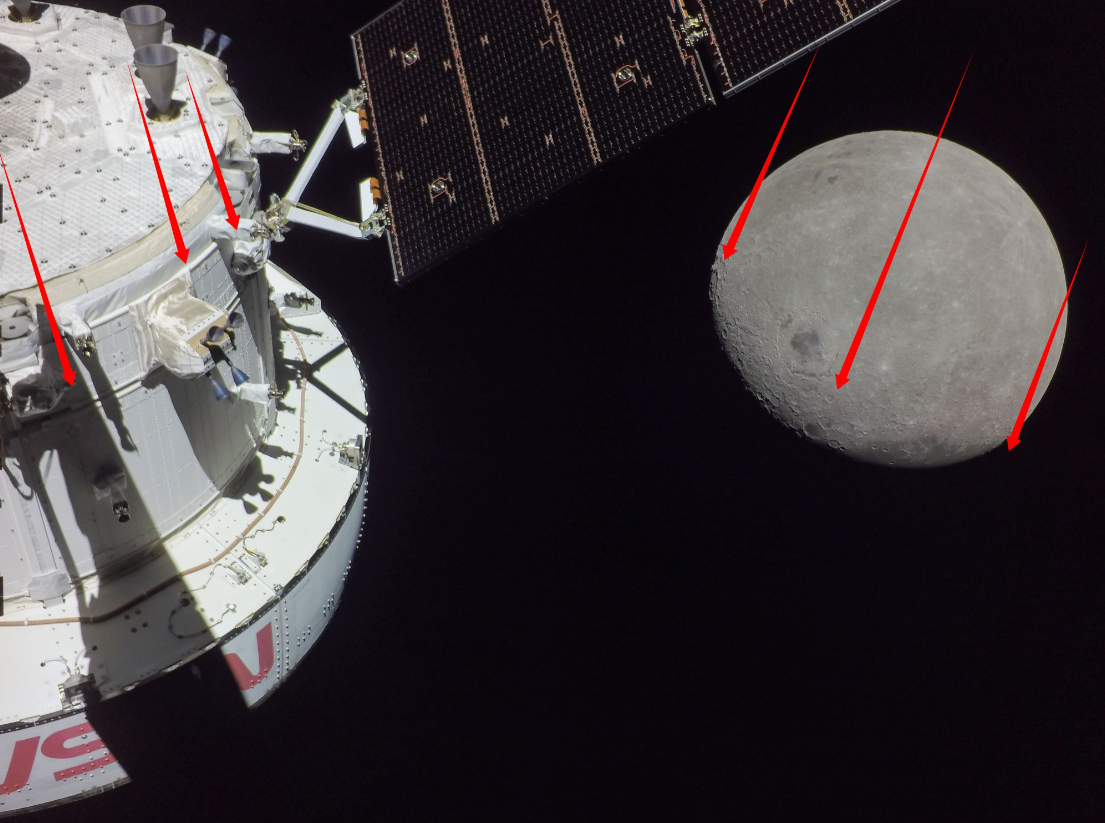
Pretty cool pic, but I can understand someone getting confused over the lighting angles and the shadows and all that. I feel that if you rotate the image the angles are more intuitive, but I know perspective is playing tricks with my mind. I was hoping if someone had a simulator or something like that that can simulate similar conditions as that in the photograph.
Pretty cool pic, but I can understand someone getting confused over the lighting angles and the shadows and all that. I feel that if you rotate the image the angles are more intuitive, but I know perspective is playing tricks with my mind. I was hoping if someone had a simulator or something like that that can simulate similar conditions as that in the photograph.